1) What is a “mathematical” knot?

In order to get started working with knots, we need to understand what mathematicians mean by the term “knots”. A “mathematical” knot is just slightly different from the knots we see and use every day.

First, take a piece of string or rope. Tie a knot in it. Now, glue or tape the ends together. You have created a mathematical knot.

The last step, joining the ends of the rope, is what differentiates mathematical knots from regular knots. The kinds of knots mathematicians work with are always formed on a closed loop (no loose ends).

Definition:
A knot is a simple closed curve in 3-dimensional space.

What does that mean? Well, a loop like the one at the left is considered a knot in mathematical knot theory (it is a simple closed curve in 3-dimensional space). In fact this knot has a special name: the unknot. The unknot can be drawn with no crossings, and is also called a trivial knot. It is the simplest of all knots.

 

2) The Central Problem of Knot Theory

The central problem of Knot Theory is determining whether two knots can be rearranged (without cutting) to be exactly alike.

A special case of this problem is one of the fundamental questions of Knot Theory: Given a knot, is it the unknot? Now, for a simple loop, that’s an easy question. But, take for example the trefoil knot animated at the top of the page. Is it possible to transform this knot so that it looks like the unknot? Tie a trefoil knot yourself and see if you can untangle it to form a simple circular loop.

When we actually start trying to untangle and rearrange knots to look like one another, we begin what can seem like a very complicated process. Mathematicians were perplexed at the seemingly unending number of ways a knot could be shaped and turned. What was needed was a simple set of rules for working with knots.

Finally, German mathematician Kurt Reidemeister (1893-1971) proved that all the different transformations on knots could be described in terms of three simple moves. The next section will give us the simple tools we need to begin working with knots in a mathematical context.

 

3) How do we work with knots? (The Reidemeister moves)

In 1926, Kurt Reidemeister (ride-a-my-stir) proved that if we have different representations (or projections) of the same knot, we can get one to look like the other using just three simple types of moves.

The Reidemeister Moves
1. Take out (or put in) a simple twist in the knot:

2. Add or remove two crossings (lay one strand over another):

3. Slide a strand from one side of a crossing to the other:

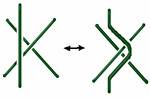
These three types of moves are called Reidemeister moves.  TheActivities Page contains knot activities that have you use these three moves to find out if two knots are indeed equivalent.

 

4) Classifying different knots

One of the first objectives mathematicians wished to achieve in working with knots was formulating tables of distinct knots. To be able to classify knots, it is easiest if we work with only one projection (or representation) of each knot to avoid duplication.

First, we must “simplify” the knot as much as possible. This means we use the Reidemeister moves (from above) to get as few crossings in the knot as possible. Once we simplify the knot so that we cannot remove any further crossings, the knot is classified by the number of crossings that remain. For example, the trefoil knot is classified by its fewest number of crossings – three (see the diagram below).

Sometimes it is possible to have more than one knot with the same number of crossings. In this case, we usually use subscripts to denote different knots with the same number of crossings, such as the 51 and52 knots in the diagram below:

The Activities Page has links to two different tables of knots classified as described above. These tables can help you see how to get started forming and working with different knots.

 

5) Properties of knots

We can change the way a knot looks so much that it can be hard to tell what we started with. So, what stays the same about a knot in different projections?

Knots have some properties that depend only on the knot itself and not on how it is looking at any particular moment. These properties are called invariants of the knot.

One invariant is the minimal crossing number. The minimal crossing number of a knot is the least number of crossings that appear in any projection of the knot. For example, the unknot has a minimal crossing number of 0. The trefoil knot has a minimal crossing number of 3. The classifications in number 4 above rely on this minimal crossing number. No matter how much we tangle a knot (without cutting), it can always be simplified to its minimal number of crossings using the Reidemeister moves.

Another invariant is the unknotting number. The unknotting number is the least number of crossing changes necessary to turn a knot into the unknot. By “crossing changes” we mean changing the orientation of two strings where they cross. The trefoil knot can be unknotted by changing only one crossing from under to over, so the trefoil knot has an unknotting number of 1 (try this to verify it).

 

6) Close cousins – Knots vs. Links

Is the figure to the right a knot?
Sometimes we run into figures that cannot be classified as knots. This figure at the right looks kind of like a knot, but we no longer have a simple closed curve – we have a group of simple closed curves (three separate loops). The new figure is called a link.

A link is a collection of knots; the individual knots which make up a link are called the components of the link. This specific link shown above is known as the Borromean Rings.

An interesting fact about the Borromean Rings: If you remove one of the component loops, the other two loops will no longer be connected! An interesting question: Can the Borromean Rings be formed using 3 flat closed loops? Not sure? Give it a try!

Just as mathematicians try to untangle knots to form the unknot, we try to separate links to form the “unlink.” A link is referred to as splittable if the component loops can be separated without cutting.

 

Once you understand the basics of what a knot is and how we tell if knots are the same, try the KT Activities Page or go straight on to Advanced KT for knot arithmetic, wild knots, and more.